One of the permeating themes in mathematics is the study of structures. When we study a mathematical object, we hope to find out something nice about it. The more structures it possesses, the nicer it mathematically is, and the more relationships to other mathematical objects it can spawn.
In this article we would like to discuss two important structures in mathematics--group structure and topological structure--and how they interact. For the definitions of groups and topological spaces, see
here and
here.
There are abundant examples of topological spaces which at the same time are group themselves. For instance,
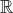
is a group with respect to addition, and a topological space, with open sets generated by open intervals. We say that the group structure is
compatible with the topological structure of
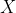
if left multiplication, right multiplication by any element, and taking inverse are continuous maps on
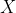
. So addition in
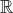
is compatible with its standard topology. This definition is crucial in our discussion for the following two reasons. First, it ties the two structures together. Second, it excludes examples of topological spaces with pathological group structures which would be uninteresting. Consider
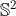
with its group multiplication defined by
+f(y)))
where
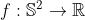
is any set-theoretic bijection. We know that
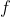
can be extremely sporadic.
Now here comes the question: given a topological space, does there always exist a compatible group structure?
Let's get our hands dirty by working out some concrete examples. We want to see if there is any compatible group structure in
)
. If you are reading this article carefully enough, you may notice that the previous discussion gives a hint to construct a suitable multiplication in
)
. Simply observe that
: (-1, 1)\to\mathbb{R})
is a homeomorphism and so it is natural to define, for
)
)
It can be easily verified that it indeed is a compatible group structure. The above construction gives a recipe to define a compatible group structure to any topological space which is homeomorphic to another known to have a compatible group structure.
Now we modify the topological space a little bit and consider
![[-1, 1]](http://www.codecogs.com/gif.latex?[-1, 1])
, the closure of
)
. It turns out that the addition of two endpoints not only alters the topology, but also makes the new space void of any compatible group structure. The reader is encouraged to prove this fact and we will discuss it in the sequel.
The above example has told us that in order for topological spaces to have compatible group structures, some topological assumptions need to be imposed.
What if the topological space is a circle
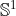
? An open disk

? A cylinder
![\mathbb{S}^1\times[-1, 1]](http://www.codecogs.com/gif.latex?\mathbb{S}^1\times[-1, 1])
?
1 則留言:
Nice post Alex! If you didn't tag the post with fixed point theorems I may not realize the connection ;p
So what do you do with the cylinder? Does every map from the (2-dimensional) closed annulus to itself has a fixed point?
張貼留言